NotesAtHKU

Pin-joined trusses

A truss is a structure that consists of straight members connected at their ends by pin joints.

A triangular truss is a statically determinate structure, but a quadrangular truss is not.

Zero-force members

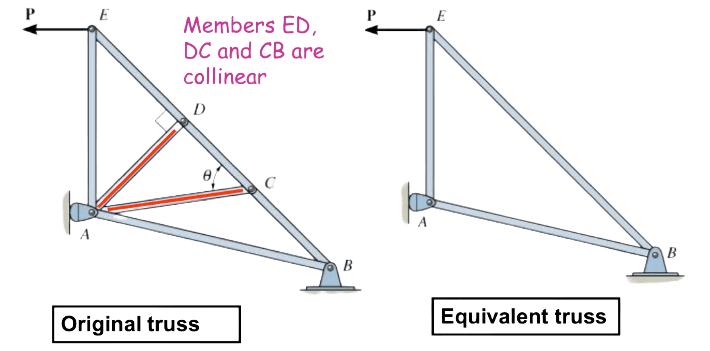
The following are zero-force members given that no external load or support reaction is applied to the joint.

2 non-collinear members of a two-member-joint
3rd member of a three-member-joint, where the other two are collinear. Additionally, members that provide no structural support against the applied load are also considered to be zero-force members.

Removing zero-force members allows us to simplify a truss structure.

Statically determinate trusses

To construct an internally statically determinate truss, we begin with a triangular pin-jointed truss and then successively adding two new members with a new joint.

We say the truss is only internally statically determinate because the external reactions are not yet known.

Truss analysis

When we analyze a truss, we assume that forces are only applied at joints.

Internal vs. External forces

Forces acting on the members $\to$ Internal forces
Forces acting on joints $\to$ External forces

Sign convention of analysis

As we focus on the analysis of internal forces acting on joints, we always draw:

Tension forces $\to$ pointing away from the joint $\{\leftarrow\circ\boxed{\rightarrow\ T\ \leftarrow}\circ\rightarrow\}$
Compression forces $\to$ pointing towards the joint $\{\rightarrow\circ\boxed{\leftarrow\ C\ \rightarrow}\circ\leftarrow\}$ During analysis, we always draw forces pointing towards the joint $\{\rightarrow\circ\leftarrow\}$ , which means we treat compression as positive.

We also treat downward forces as positive $\{+\downarrow\}$ .

Equilibrium sections

If we cut out any section of an equilibrium system, the cut will be in equilibrium. That is, the external forces are balanced by internal forces.

Therefore, to solve for the forces in a system, we simply cut out a section that we deem solvable (by practice!) and draw it's FBD. The forces includes all external forces in the cut as well as the interal forces of the cut members.

Note that to solve for a joint, the number of unknowns must be equal to the number of equations of equilibrium.

Cable anaylsis

Cable forces

Cables are always in tension. The tension force is always in the direction of the cable.

Some characteristics of cable structures:

Supports are always 2 inverted pinned supports
Applied forces all point downwards due to gravity
Horizontal reaction forces at supports are equal in magnitude

Structural analysis